|
|
|
|
Next: 19.1.2 More on delta and Green’s functions |
|
Question:
Show that
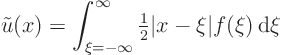
Answer:
First of all, define a second anti-derivative of ![]() to be
to be ![]() . That allows you from now on to write
. That allows you from now on to write ![]() as
as ![]() . Note also that
. Note also that ![]() is one possible solution to the Poisson equation. The most general solution is this particular solution plus the general solution of the homogeneous equation. Use your knowledge of ordinary differential equations to show that the most general solution is
is one possible solution to the Poisson equation. The most general solution is this particular solution plus the general solution of the homogeneous equation. Use your knowledge of ordinary differential equations to show that the most general solution is
Now restrict the region of integration of ![]() to
to ![]()
![]()
![]()
![]()
![]() where
where ![]() is some large number. Take
is some large number. Take ![]()
![]()
![]() so that the integral is zero beyond
so that the integral is zero beyond ![]() . Also, take
. Also, take ![]() large enough that the particular
large enough that the particular ![]() at which you want to find
at which you want to find ![]() is in the range
is in the range ![]()
![]()
![]()
![]()
![]() . Show that that since
. Show that that since ![]() is zero for
is zero for ![]()
![]()
![]() ,
,
Split the integral into two parts ![]()
![]()
![]() and
and ![]()
![]()
![]() because the absolute value in the integral is different in these two cases. You get
because the absolute value in the integral is different in these two cases. You get
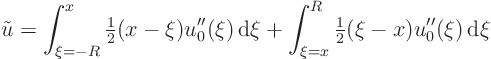
You then find that ![]() is indeed a solution to the Poisson equation.
is indeed a solution to the Poisson equation.