18.2.2.2 Solution stanexh-b

Question:

This is a continuation of a corresponding question in the subsection on the Laplace equation. See there for a definition of terms.

Derive the heat equation for unsteady heat conduction using vector analysis.

Answer:

The amount of thermal energy residing in a given volume is equal to

$$\int\rho C_p u { \rm d}V$$

plus a constant that is not important.

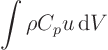
The time derivative of this energy is of course the net heat energy flowing in per unit time. Which is minus the heat energy flowing out. That heat flow was derived in the earlier section. Use the divergence theorem to convert it into a volume integral.

Put the two together to get the heat equation. Note that if a volume integral is zero regardless of what you take the volume to be, the integrand must be zero.