so that
. If A is square and |A| is nonzero, the null space
is simply 
and has dimension 0.
The null space of a matrix A are all vectors so that
. If A is square and |A| is nonzero, the null space
is simply and has dimension 0.
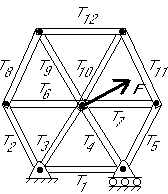
Nontrivial null spaces may correspond to internal stresses in structures, connectivity problems, vibrational mode shape, buckling shapes, eigenvectors corresponding to a given eigenvalue, etcetera.
You typically want to describe the null spaces as simply as possible. Defining a basis for the null space allows you to do so.
09/27/02 0:27:37 09/30/02 0:00:19