§3 Rotation

For linear, constant coefficient equations, rotate the coordinate system to the principal axes of A:

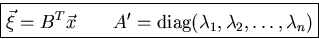
provided that B consists of the orthonormal eigenvectors of A.

Our equation simplifies to

Notes:

1.

If A is not constant, we must select a point P for which we determine the eigenvectors. The new A' will then only be diagonal at the point P.

2.

If A is not constant, trying to set B at every point equal to the eigenvectors of the A at that point will not usually work since it requires n² equations to be satisfied by the n components of .

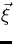3.

If we do not normalize the eigenvectors,