7.36, §5 Back

We need to find the original to

Looking in the tables:

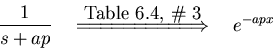
The other factor is a shifted function f, restricted to the interval that its argument is positive:

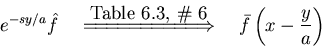
With the bar, I indicate that I only want the part of the function for which the argument is positive. This could be written instead as

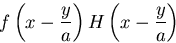
where the Heaviside step function H(x)=0 if x is negative and 1 if it is positive.

Use convolution, Table 6.3, # 7. again to get the product.

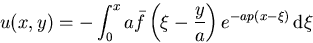
This must be cleaned up. I do not want bars or step functions in my answer.

I can do that by restricting the range of integration to only those values for which 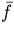is nonzero. (Or H is nonzero, if you prefer)

Two cases now exist:

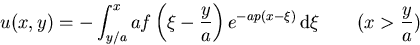
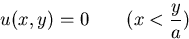
It is neater if the integration variable is the argument of f. So, define 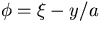and convert:

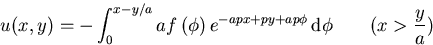
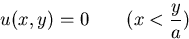
This allows me to see which physical f values I actually integrate over when finding the flow at an arbitrary point: