2 01/24 F

1. 1st Ed: p78, q46, 2nd Ed: p91, q46. $r=\sqrt{x^2+y^2+z^2}$

2. 1st Ed: p78, q54, 2nd Ed: p92, q54. You may want to refresh your memory on total derivatives.

3. 1st Ed: p78, q60, 2nd Ed: p92, q60. (20 points) Also find two scalar equations that describe the line through P that crosses the surface normally at P.

   Find the unit normal $\vec n$ to the surface at P. Now assume that the surface is reflective, satisfying Snell's law. An incoming light beam parallel to the $x$-axis hits the surface at P. Find a vector equation that describes the path of the reflected beam.

   Hint: let $\vec v$ be a vector along the light ray. The component of $\vec v$ in the direction of $\vec n$ is $\vec n\cdot\vec v$. The component vector in the direction of $\vec n$ is defined as $\vec v_1=\vec n(\vec n\cdot\vec v)$. Sketch this vector along with vector $\vec n$. In which direction is the remainder $\vec v_2 =\vec v - \vec v_1$? Now figure out what happens to $\vec v_1$ and $\vec v_2$ during the reflection. Take it from there.

4. 1st Ed: p79, q64, 2nd Ed: p92, q64.

5. 1st Ed: p80, q87, 2nd Ed: p93, q87. (20 points) Compare with a point sink in which
   
   $$\vec v = -\frac{x{\hat\imath}+y{\hat\imath}}{x^2+y^2}$$
   
   
   Assume these are incompressible flows, in which the fluid density is constant. For each flow, compute the divergence, draw streamlines, and figure out how much fluid passes through a circle of arbitrary radius $r$. (Since the velocity is radial, the fluid flow through a circle is the magnitude of the velocity times the circumference of the circle.) Now look at a ring between two slightly different radii, and compare the fluid that goes in at one radius with the fluid that goes out at the other radius. 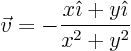Based on the results, argue that the divergence of the velocity is a measure of the source strength, the amount of fluid created out of nothing. (A sink being a negative source, where fluid disappears into nothing.) So, what do you think of the value of the divergence of the point sink at the origin (assuming that you smooth out the singularity a bit)? Note: if the fluid is not incompressible, it is really volume flows you are comparing, not mass flows, and the divergence is a measure of the relative rate of specific volume expansion. Additional volume is created out of nothing, not mass.

6. 1st Ed: p80, q102, 2nd Ed: p94, q102. Make sure that you find $\phi$ in a mathematically sound way, as discussed in class. No messing around and guessing a solution!