Reynolds Decomposition

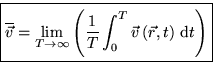
(Book 23.3, 4)

Decompose the flow quantities in average and fluctuating components. Time average:

Fluctuating part:

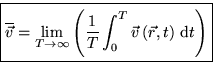
Reynolds averaged equations can be found by averaging the Navier Stokes equations:

Continuity

Now from the definition of time average, the time average of a time average leaves that time average unchanged. Also the time average of any fluctuating component is zero. Further, the time average is a linear operation and commutes with differentiation, so

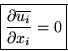
Viscous Stress Tensor: Assuming is constant,

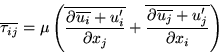
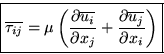
Momentum Equations: Using the conservation form,

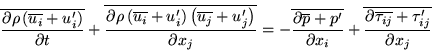
It is seen that the turbulent velocity fluctuations act as an additional shear stress called the Reynolds stress:

Unfortunately, the Reynolds stress is not known unless you solve the full unsteady Navier-Stokes equations. To avoid this humongous task, guessing (also known as modelling) is needed.

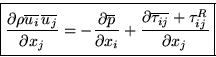
Molecular model of (laminar) viscosity:

(Book 6.2)

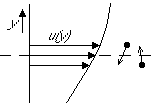
Consider the net momentum transfer per unit area through the plane indicated by the broken line. This momentum transfer produces the shear stress. Molecules that cross the plane travelled towards the plane over a distance on the order of a mean free path length without colliding. So the molecules reaching the plane from above have an average x-velocity that is roughly higher than the average u at the plane, decreasing the x momentum above the broken line. The fluid particles that reach the plane from below have an average x-velocity that is roughly lower than the average u at the plane, also decreasing the x momentum above the broken line. As a result, the fluid above the plane is slowed down at the plane. The force per unit area follows as the part of that not cancels between the downward and upward moving molecules. The crossing velocity corresponding to is of the order of the mean molecular speed, which is of the order of the speed of sound a. So the stress is of order , and the kinematic viscosity of order .

Mixing length idea:

Assume that the turbulent transport of momentum is similar to the molecular one. Fluid at a given plane originates from some transverse distance with much of its velocity difference left intact during the trip. Then the kinematic eddy viscosity would be , where v' is the typical vertical velocity fluctuation. From continuity, u'_x + v'_y = 0, so assuming that there is no strong directionality in eddy length scales, v' is of the order u', which was estimated as , giving an eddy viscosity

Assuming is a known quantity, this gives governing equations that are partial differential equations. The estimate of could be the typical transverse length scale in free turbulence or the distance from the wall in a surface layer.

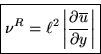
Many things are wrong in the story: For one, turbulent motion is not small compared to the transverse scales of the flows, so and is in fact related to the velocity at finite distances, making the entire idea of having universal partial differential equations (involving local quantities only) suspect. Also, the turbulent fluctuations are not independent of the velocity field like a, the turbulent shear stress would always be predicted to be exactly zero at points of even if there is no symmetry around that point, the larger turbulence scales are directional, etc.

04/14/00 0:22:36 04/14/00 0:24:35 04/19/00 0:15:43