A.44 Rel­a­tivis­tic neu­tri­nos

It is cer­tainly du­bi­ous to de­scribe beta de­cay non­rel­a­tivis­ti­cally. Neu­tri­nos are highly rel­a­tivis­tic par­ti­cles with al­most zero rest mass. So [16, p. 258] rightly states that it is ab­surd to treat them non­rel­a­tivis­ti­cally. Then he im­me­di­ately pro­ceeds to do it any­way. (But he con­firms the re­sults later us­ing a proper analy­sis.)

One big prob­lem is that rel­a­tivis­ti­cally, spin and or­bital an­gu­lar mo­men­tum be­come mixed-up. That prob­lem was en­coun­tered ear­lier for the pho­ton, which is an even more rel­a­tivis­tic par­ti­cle. Chap­ter 7.4.3 needed some con­tor­tions to talk around that prob­lem.

But note that while the prob­lem for the neu­trino is qual­i­ta­tively sim­i­lar, the de­tails are quite dif­fer­ent. While the pho­ton is a bo­son, the neu­trino is a fermion. Fermi­ons, un­like bosons, are de­scribed by the Dirac equa­tion, chap­ter 12.12 ver­sus {A.21}.

To un­der­stand the Dirac equa­tion re­quires some lin­ear al­ge­bra, in par­tic­u­lar ma­tri­ces. But to un­der­stand the dis­cus­sion here, the in­for­ma­tion in the no­ta­tions sec­tion should be plenty.

Con­sider first the Dirac Hamil­ton­ian eigen­value prob­lem for the elec­tron and its an­tipar­ti­cle, the positron, in empty space. It is sim­plest thought of as a sys­tem of two equa­tions:

$$\fbox{$\displaystyle m c^2 \vec\psi^- + c {\skew 4\widehat... ...ew{-.5}\vec p}}\cdot\vec\sigma \vec\psi^- = E \vec\psi^+ $} %$$ (A.271)

Here $c$ is the speed of light and $m$ the rest mass of the elec­tron or positron. So $mc^2$ is the rest mass en­ergy ac­cord­ing to Ein­stein’s fa­mous re­la­tion. Fur­ther ${\skew 4\widehat{\skew{-.5}\vec p}}$ is the lin­ear mo­men­tum op­er­a­tor. And $\vec\sigma$ is the spin an­gu­lar mo­men­tum op­er­a­tor, ex­cept for a scale fac­tor. More pre­cisely, $\vec\sigma$ $\vphantom0\raisebox{1.5pt}{$=$}$ ${\skew 6\widehat{\vec S}}$$\raisebox{.5pt}{$/$}$​$\frac12\hbar$. The three com­po­nents of $\vec\sigma$ are the fa­mous Pauli spin ma­tri­ces.

In the non­rel­a­tivis­tic limit, $\vec\psi^-$ be­comes the wave func­tion of an elec­tron. Re­call from chap­ter 5.5.1 that this wave func­tion can be thought of as a vec­tor with two com­po­nents; the first com­po­nent cor­re­sponds to the spin-up state and the sec­ond to the spin-down state. Sim­i­larly $\vec\psi^+$ be­comes the wave func­tion of a positron.

The non­rel­a­tivis­tic limit means math­e­mat­i­cally that the rest mass en­er­gies $mc^2$ are much larger than the ki­netic en­er­gies. Or more sim­ply, it is the limit $c\to\infty$. Un­der those con­di­tions, by ap­prox­i­ma­tion,

$$m c^2 \vec\psi^- \approx E \vec\psi^- \qquad - m c^2 \vec\psi^+ \approx E \vec\psi^+$$

So in the non­rel­a­tivis­tic limit the en­ergy of the elec­tron is ap­prox­i­mately the rest mass en­ergy, as it should be. Note how­ever that the value of $E$ for a positron is neg­a­tive. That should not be taken to mean that the rest mass en­ergy of a positron is neg­a­tive. It is the same as the one of an elec­tron, pos­i­tive. The positron is an an­tipar­ti­cle, and the value of $E$ of an an­tipar­ti­cle picks up an ex­tra mi­nus sign be­cause these par­ti­cles move back­ward in time. Quan­tum me­chan­ics re­lates $E$ to the time de­riv­a­tive as $E\to{\rm i}\hbar\partial/\partial{t}$, as seen in the Schrö­din­ger equa­tion, so a sign change in $E$ is equiv­a­lent to a re­ver­sal in time.

If you im­prove the ac­cu­racy of the non­rel­a­tivis­tic ap­prox­i­ma­tions a bit us­ing some math­e­mat­i­cal ma­nip­u­la­tion, the en­ergy will also in­clude the clas­si­cal ki­netic en­ergy. To see how that works, (with­out us­ing more proper ma­trix eigen­value meth­ods), pre­mul­ti­ply the sec­ond equa­tion in (A.271) by ${\skew 4\widehat{\skew{-.5}\vec p}}\cdot\vec\sigma$$\raisebox{.5pt}{$/$}$​$2mc$ and add it to the first. In do­ing so, note that $({\skew 4\widehat{\skew{-.5}\vec p}}\cdot\vec\sigma)^2$ is sim­ply ${\widehat p}^{ 2}$. That can be ver­i­fied by mul­ti­ply­ing out the Pauli ma­tri­ces given in chap­ter 12.10. In par­tic­u­lar,

$$({\skew 4\widehat{\skew{-.5}\vec p}}\cdot\vec\sigma)({\skew... ...j+\sigma_j\sigma_i = 0 \mbox{ if } i\ne j \end{array} \right.$$

Tak­ing that into ac­count, you get

$$\left(m c^2 + \frac{{\widehat p}^{ 2}}{2m}\right) \vec\psi... ...at{\skew{-.5}\vec p}}\cdot\vec\sigma}{2mc^2} \vec\psi^+\right)$$

This ex­pres­sion can be rewrit­ten as

$$\left(m c^2 + \frac{{\widehat p}^{ 2}}{2m}\right) \left(\v... ...t{\skew{-.5}\vec p}}\cdot\vec\sigma}{2mc^2} \vec\psi^+ \right)$$

(If you mul­ti­ply this out, you get an ad­di­tional term, but it is neg­li­gi­bly small com­pared to the rest.) Now note that this is an eigen­value prob­lem for an elec­tron whose wave func­tion has picked up a lit­tle bit of $\vec\psi^+$. You might say that the slightly rel­a­tivis­tic elec­tron picks up a bit of a non­rel­a­tivis­tic positron wave func­tion. Also its en­ergy has picked up an ad­di­tional term ${\widehat p}^{ 2}$$\raisebox{.5pt}{$/$}$​$2m$, the clas­si­cal ki­netic en­ergy.

De­riva­tion {D.81} shows how take this fur­ther, and do it rig­or­ously us­ing proper lin­ear al­ge­bra pro­ce­dures.

But this ad­den­dum is not re­ally in­ter­ested in the non­rel­a­tivis­tic limit. In­stead it is in­ter­ested in the ul­tra­rel­a­tivis­tic limit, where it is the rest mass that is neg­li­gi­ble com­pared to the ki­netic en­ergy. And for zero rest mass, the Dirac eigen­value prob­lem (A.271) be­comes

$$c {\skew 4\widehat{\skew{-.5}\vec p}}\cdot\vec\sigma \vec\p... ...{\skew{-.5}\vec p}}\cdot\vec\sigma \vec\psi^- = E \vec\psi^+ %$$ (A.272)

To make some sense out of this, de­fine two new par­tial wave func­tions as

$$\vec\psi_{\rm {R}} = \frac{\vec\psi^- + \vec\psi^+}{2} \qquad \vec\psi_{\rm {L}} = \frac{\vec\psi^- - \vec\psi^+}{2}$$

By tak­ing sums and dif­fer­ences of the ul­tra­rel­a­tivis­tic equa­tions above, you then get

$$\fbox{$\displaystyle c {\skew 4\widehat{\skew{-.5}\vec p}}... ...}\cdot\vec\sigma \vec\psi_{\rm{L}} = E \vec\psi_{\rm{L}} $} %$$ (A.273)

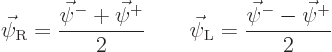
The math­e­mati­cian Weyl first noted how re­mark­able these equa­tions re­ally are. The equa­tions for the two par­tial wave func­tions are not cou­pled! Par­tial wave func­tion $\vec\psi_{\rm {L}}$ is not in the equa­tion for $\vec\psi_{\rm {R}}$ and $\vec\psi_{\rm {R}}$ is not in the one for $\vec\psi_{\rm {L}}$. So it seems like each equa­tion might de­scribe a par­ti­cle whose wave func­tion is a sim­ple two-di­men­sion­al vec­tor. In other words, each equa­tion could de­scribe a par­ti­cle with­out a part­ner. Note in­ci­den­tally that the sec­ond equa­tion is the neg­a­tive $E$ ver­sion of the first. So pre­sum­ably the sec­ond equa­tion could merely de­scribe the an­tipar­ti­cle of the par­ti­cle of the first equa­tion.

Rea­son­ably speak­ing, these par­ti­cles should be elec­tri­cally neu­tral. Af­ter all, their wave func­tions are equal com­bi­na­tions of $\vec\psi^-$, the equiv­a­lent of the neg­a­tively charged non­rel­a­tivis­tic elec­tron in this sys­tem, and $\vec\psi^+$, the equiv­a­lent of the pos­i­tively charged non­rel­a­tivis­tic positron. So these par­ti­cles should be neu­tral as well as mass­less. In short, they should be neu­tri­nos.

There are a cou­ple of prob­lems how­ever. The Dirac equa­tion in­cludes both the elec­tron and the positron, the time-re­versed elec­tron. If you write an equa­tion for a par­ti­cle that does not in­her­ently in­clude a time-re­versed part­ner, are you vi­o­lat­ing time-re­ver­sal sym­me­try?

The other prob­lem is what hap­pens if you look at na­ture in the mir­ror. Or rather, what you re­ally want to do is in­ver­sion: swap the pos­i­tive di­rec­tion of all three axes in a Carte­sian co­or­di­nate sys­tem. This has the ef­fect of cre­at­ing a mir­ror im­age of na­ture, since the co­or­di­nate sys­tem is now left-handed in­stead of right-handed. And its ef­fects are math­e­mat­i­cally eas­ier to de­scribe.

First con­sider what hap­pens to the orig­i­nal Dirac equa­tion (A.271). The lin­ear mo­men­tum op­er­a­tor ${\skew 4\widehat{\skew{-.5}\vec p}}$ in­tro­duces a mi­nus sign un­der in­ver­sion. That is be­cause this op­er­a­tor is pro­por­tional to the gra­di­ent op­er­a­tor $\nabla$, and every Carte­sian co­or­di­nate in $\nabla$ changes sign. How­ever, the spin op­er­a­tors $\vec\sigma$ are like or­bital an­gu­lar mo­men­tum, ${\skew0\vec r}$ $\times$ ${\skew 4\widehat{\skew{-.5}\vec p}}$, so they in­tro­duce two mi­nus signs, which means no sign change.

(These ar­gu­ments as pre­sented look at the dot prod­uct purely al­ge­braically. If you con­sider the gra­di­ent as a vec­tor, there is an ad­di­tional sign change since the unit vec­tors change di­rec­tion. But the same holds for the $\vec\sigma$ vec­tor in the dot prod­uct, so there is no dif­fer­ence in the fi­nal an­swer.)

At face value then, the ${\skew 4\widehat{\skew{-.5}\vec p}}\cdot\vec\sigma$ terms in the Dirac sys­tem change sign. That would mean that na­ture does not be­have in the same way when seen in the mir­ror; the Dirac equa­tion is not the same. And that is def­i­nitely wrong; it is very ac­cu­rately es­tab­lished that elec­tro­mag­netic sys­tems in­volv­ing elec­trons do be­have in ex­actly the same way when seen in the mir­ror.

For­tu­nately there is a loop­hole to save the math­e­mat­ics of the Dirac equa­tion. It can be as­sumed that ei­ther the elec­tron or the positron has neg­a­tive in­trin­sic par­ity. In other words it can be as­sumed that ei­ther non­rel­a­tivis­tic wave func­tion changes sign un­der in­ver­sion. You can check that if ei­ther $\vec\psi^-$ or $\vec\psi^+$ changes sign un­der in­ver­sion, then the Dirac sys­tem stays the same un­der in­ver­sion. Which one of the two changes sign is not im­por­tant, since the sign of the wave func­tion does not make a dif­fer­ence for the physics. It is con­ven­tion to as­sume that the an­tipar­ti­cles of fermi­ons have the neg­a­tive in­trin­sic par­ity. In that case, you do not need to worry about in­trin­sic par­ity if you have no an­tipar­ti­cles present.

But now re­con­sider the Hamil­ton­ian eigen­value prob­lem (A.273) for the neu­tri­nos. The trick no longer works! If you can have one of these par­ti­cles by it­self, not tied to a part­ner, the physics is no longer the same when seen in the mir­ror.

There is an im­por­tant con­se­quence to that. If na­ture is the same when seen in the mir­ror, there is a con­served math­e­mat­i­cal quan­tity called par­ity. That is just like there is a con­served quan­tity called an­gu­lar mo­men­tum be­cause na­ture be­haves in the same way when you look at it in a ro­tated co­or­di­nate sys­tem, chap­ter 7.3. What it boils down to is that Weyl was say­ing that par­ity might not be con­served in na­ture.

At the time, Pauli lam­basted Weyl for dar­ing to sug­gest some­thing so ob­vi­ously stu­pid like that. How­ever, Pauli lived long enough to learn in 1956 that ex­per­i­ments showed that in­deed neu­tri­nos do not con­serve par­ity. (Weyl had al­ready died.)

The fact that the Weyl Hamil­to­ni­ans do not con­serve par­ity can be de­scribed more ab­stractly. Let ${\mit\Pi}$ be the “par­ity op­er­a­tor” that ex­presses what hap­pens to a wave func­tion when the axes are in­verted. The above dis­cus­sion can then be sum­ma­rized ab­stractly as

$${\mit\Pi}(c{\skew 4\widehat{\skew{-.5}\vec p}}\cdot\vec\sig... ...\widehat{\skew{-.5}\vec p}}\cdot\vec\sigma) {\mit\Pi}\vec\psi$$

In words, when you in­vert axes, the ${\skew 4\widehat{\skew{-.5}\vec p}}\cdot\vec\sigma$ op­er­a­tor in­tro­duces a mi­nus sign. Also the wave func­tion $\vec\psi$ changes into its mir­ror im­age. Now the ex­pres­sion in paren­the­ses is the first Weyl Hamil­ton­ian, or mi­nus the sec­ond. So the ex­pres­sion above can be read that if you in­ter­change the or­der of the par­ity op­er­a­tor and ei­ther Hamil­ton­ian, it in­tro­duces a mi­nus sign. It is said that the par­ity op­er­a­tor and the Hamil­ton­ian do not com­mute: the or­der in which they are ap­plied makes a dif­fer­ence.

It is quite gen­er­ally true that if an op­er­a­tor does not com­mute with the Hamil­ton­ian, the cor­re­spond­ing quan­tity is not pre­served. See for ex­am­ple chap­ter 4.5.1, 7.1.4 and {A.19}. So you might ask whether or­bital an­gu­lar mo­men­tum is pre­served by the Weyl Hamil­ton­ian. If you grind out the com­mu­ta­tor of an or­bital an­gu­lar mo­men­tum com­po­nent with the Weyl Hamil­ton­ian us­ing the rules of chap­ters 4.5.4 and 5.5.3, you find that you get some­thing nonzero. So or­bital an­gu­lar mo­men­tum is not con­served by the Weyl neu­tri­nos. That was no big sur­prise to physi­cists, be­cause the Dirac equa­tion does not con­serve or­bital an­gu­lar mo­men­tum ei­ther. Sim­i­larly, if you work out the com­mu­ta­tor of a com­po­nent of spin, you find that spin is not pre­served ei­ther.

How­ever, if you add the two op­er­a­tors, you get the net an­gu­lar mo­men­tum, or­bital plus spin. That op­er­a­tor does com­mute with the Weyl Hamil­to­ni­ans. So the Weyl Hamil­to­ni­ans do con­serve net an­gu­lar mo­men­tum, like the Dirac equa­tion does. That is very for­tu­nate, be­cause with­out doubt Pauli would have had a fit if Weyl had sug­gested that na­ture does not con­serve net an­gu­lar mo­men­tum.

But rel­a­tiv­ity does mix up or­bital an­gu­lar mo­men­tum with spin. Of­ten this book de­scribes par­ti­cles as be­ing in states of spe­cific or­bital an­gu­lar mo­men­tum with spe­cific val­ues of the spin. For ex­am­ple, that is how the non­rel­a­tivis­tic hy­dro­gen atom was de­scribed, chap­ter 4.3. Such a de­scrip­tion is not re­ally jus­ti­fied for par­ti­cles at rel­a­tivis­tic speeds. For­tu­nately the elec­tron in a hy­dro­gen atom has a ki­netic en­ergy much less than its rest mass en­ergy. Things are much worse in beta de­cay, where the neu­trino is emit­ted at al­most the speed of light.

You might ask what else the Weyl Hamil­to­ni­ans com­mute with. It can be seen that they com­mute with the lin­ear mo­men­tum op­er­a­tors: dif­fer­ent lin­ear mo­men­tum op­er­a­tors com­mute be­cause ba­si­cally they are just de­riv­a­tives, and spin com­mutes with non­spin. Dif­fer­ent spin com­po­nents do not com­mute, but every­thing com­mutes with it­self. Since the Hamil­ton­ian only in­volves the spin com­po­nent in the di­rec­tion of the lin­ear mo­men­tum, (be­cause of the dot prod­uct), the Hamil­ton­ian com­mutes with the spin in that di­rec­tion.

It is in­struc­tive to form a men­tal pic­ture of these neu­tri­nos. Ac­cord­ing to the above, the neu­tri­nos can be in states with def­i­nite lin­ear mo­men­tum and with def­i­nite spin in the di­rec­tion of that lin­ear mo­men­tum. In those states the neu­tri­nos move in a spe­cific di­rec­tion and also ro­tate around an axis in the same di­rec­tion. You can think of it macro­scop­i­cally as screws; screws too ro­tate around their axis of mo­tion while they move in or out of their hole.

Now there are two kinds of screws. Nor­mal, right-handed, screws move into the hole if you ro­tate them clock­wise with a screw­driver. Some spe­cial ap­pli­ca­tions use left-handed screws, which are ma­chined so that they move into the hole when you ro­tate them counter-clock­wise. (One of the screws that keep the ped­als on a bi­cy­cle is nor­mally a left-handed screw, to pre­vent you from loos­en­ing it up when ped­al­ing.)

Ex­per­i­ments show that all an­ti­neu­tri­nos ob­served in a nor­mal lab set­ting act like right-handed screws. Neu­tri­nos act like left-handed screws. Since a right-handed screw turns into a left-handed screw when seen in a mir­ror, na­ture is not the same when seen in a mir­ror. If, say, a beta de­cay is ob­served in a mir­ror, the an­ti­neu­trino that comes out is left-handed, rather than right-handed as it should.

(Us­ing ar­gu­ments like in {D.70}, it can be seen that $\vec\psi_{\rm {R}}$ above is righthanded and $\vec\psi_{\rm {L}}$ left handed, [53, p. 95].)

Note that the­o­ret­i­cal physi­cists do not know about screws. So they came up with a dif­fer­ent way to ex­press that an­ti­neu­tri­nos are right-handed like nor­mal screws, while neu­tri­nos are left-handed. They call the com­po­nent of spin in the di­rec­tion of mo­tion, scaled so that its val­ues are $\pm1$, the “he­lic­ity.” So ac­cord­ing to the­o­ret­i­cal physi­cists, an­ti­neu­tri­nos as seen in a lab have he­lic­ity 1, while neu­tri­nos have he­lic­ity $\vphantom{0}\raisebox{1.5pt}{$-$}$1.

It is ironic that Pauli in turn did not live long enough to learn that Weyl’s neu­tri­nos were wrong on at least one ma­jor count. Around 1998, ex­per­i­ments found that neu­tri­nos and an­ti­neu­tri­nos are not mass­less as was long thought. Their mass is ex­tremely tiny, (far too tiny to be ac­cu­rately mea­sured by ex­per­i­men­tal meth­ods avail­able at the time of writ­ing), but it is not zero.

Fun­da­men­tally, it makes a big dif­fer­ence, be­cause then their speed must be less than the speed of light. Hy­po­thet­i­cally, an ob­server can then move with a speed faster than some an­ti­neu­trino pro­duced in a lab. The an­ti­neu­trino then seems to go back­ward for that ob­server. Since the spin is still in the same di­rec­tion, the ob­server sees a left-handed an­ti­neu­trino, not a right-handed one. So the hand­ed­ness, or he­lic­ity, of an­ti­neu­tri­nos, and sim­i­larly neu­tri­nos, is not fun­da­men­tal. A process like the beta de­cay of an nu­cleus mov­ing at a speed ex­tremely close to the speed of light could pro­duce a left-handed an­ti­neu­trino trail­ing the nu­cleus. But since this does not hap­pen for a nu­cleus at rest, na­ture is still not the same when seen in the mir­ror.

At the time of writ­ing, the pre­cise na­ture of neu­tri­nos is not yet fully un­der­stood. Usu­ally, it is as­sumed that neu­tri­nos are dis­tinct from an­ti­neu­tri­nos. Neu­tri­nos for which that is true are called Dirac neu­tri­nos. How­ever, so-called Ma­jo­rana neu­tri­nos would be their own an­tipar­ti­cles; neu­tri­nos and an­ti­neu­tri­nos would sim­ply dif­fer in the spin state.