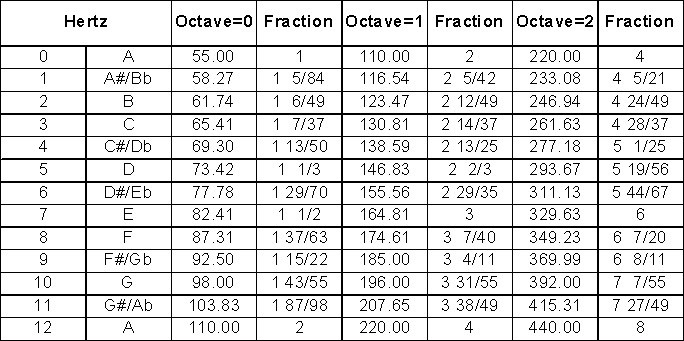
Musical Notes

The baseline for musical notes is the fundamental ‘A’, which occurs at a frequency of 55 Hz. When this frequency is doubled, i.e. 110 Hz, an octave is defined. This is the next frequency when the note played will be in harmony with the fundamental ‘A’. The octave from one note to another is then divided into 12 notes, each with a frequency difference of Hz.

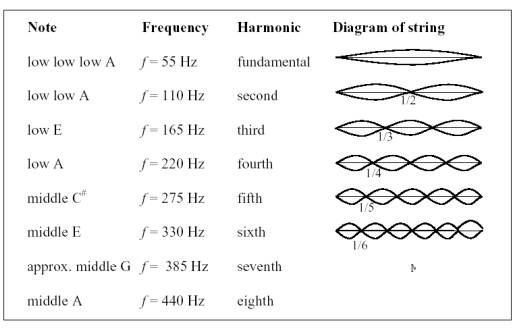When specific notes are played on instruments, they contain additional frequencies such as integer multiple components of its fundamental frequency. This phenomenon explains why the same note played on different instruments has different sounds. For instance, when looking at the Fourier transform of a note played by a guitar, there are 3 frequencies that contribute to the overall sound of the note with both the second and third frequencies as integer multiples of the first. A saxophone note, on the other hand, is a combination of more than 5 frequencies, which explains the difference in sound between the two instruments. The sound produced by the addition of these harmonic waves is known as the principle of superposition.