Introduction
Cryogenic helium-4 has long been recognized as a useful fluid material for turbulence research and for cooling scientific and industrial facilities. Helium-4 has three fluid phases (see Fig. 1 (a)): a gas phase, a normal liquid phase (He-I), and a superfluid phase (He-II). The gaseous helium and He-I are classical Navier-Stokes viscous fluids [1]. He-II, on the other hand, is a quantum liquid consisting of two intermiscible fluids: a viscous normal-fluid component (i.e. the thermal excitations) whose fraction drops from unity to zero as the temperature decreases from the phase transition temperature (about 2.17 K) to zero, and an inviscid superfluid component (i.e. the condensate).
Being classical fluids, gaseous helium and He I have many unique properties that distinct them from other fluid materials. For instance, the critical point of helium-4 (Pc = 2.24 bar, Tc = 5.19 K) is easily accessible. By adjusting the pressure and temperature around the critical point, one can vary the helium density and hence its kinematic viscosity in a wide range as shown in Fig.1 (b). This property enables the generation of natural convection flows in helium with extremely high Rayleigh numbers (Ra). Studying the scaling laws of the flow parameters at large Ra numbers, where turbulent convection sets in, is of both theoretical and practical significance [2-3]. Close to its critical point, helium-4 not only allows for high Ra flows but also an incomparable 12-decade tuning range of Ra, which makes laboratory study of ocean convection and atmospheric circulation possible [2-3].
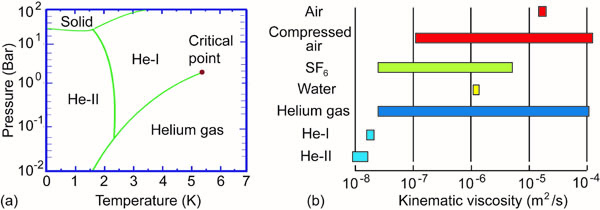
Figure 1: (a) Phase diagram of helium-4; (b) Range of kinematic viscosity of test fluids [3].
The hydrodynamics of He II is strongly affected by quantum effects. For instance, The rotational motion of the superfluid component can occur only with the formation of topological defects in the form of vortex lines [4]. These vortex lines all have identical cores with a radius of about 1 Å, and they each carry a single quantum of circulation κ=h/m4, where h is Planck’s constant and m4 is the mass of a 4He atom. Turbulence in the superfluid therefore takes the form of an irregular tangle of vortex lines (quantum turbulence) [5]. The normal fluid is expected to behave more like a classical fluid. But a force of mutual friction between the two fluids, arising from the scattering of thermal excitations by the vortex lines, can affect the flows in both fluids. He II supports the most efficient heat-transfer mechanism (i.e. thermal counterflow), and it also allows the generation of flows with extremely high Reynolds (Re) numbers for turbulence modelling due to its small kinematic viscosity. Our current research mainly focuses on the study of the fascinating quantum hydrodynamics in He II.
Despite the great potential of helium-4, a grand challenge in this field is the lack of reliable quantitative flow measuring tools. Typical single-point diagnostic tools such as pitot pressure tubes and hot-wire anemometers have limited spatial resolution, and in He-II their responses can be affected by the motion of both fluid components. When the two fluids have different velocity fields, data analysis can become impractical. Another desirable route for probing the flow is via direct flow visualization. In the past, a number of labs (include us) used micron-sized solidified particles as tracers and developed particle image velocimetry (PIV) and particle tracking velocimetry (PTV) techniques for He II [6]. These micron-sized tracers can easily get trapped on quantized vortices due to their large size and hence large binding energy to the vortex cores. The trapped tracers can yield interesting images of the vortex lines [7]. Nevertheless, the heating accompanying the tracer injection can cause violent disturbance in helium. More problematically, the strong interaction of the tracers with both the viscous normal fluid and the quantized vortices makes their motion hardly analyzable in practical turbulent flows.
Our cryogenics lab aims to develop and implement transformative novel flow visualization techniques to unlock the full potential of helium-4. Especially, in recent years we have conducted a series of experiments to successfully demonstrate the superior properties of metastable He2* molecular tracers [8]. These tracers unambiguously track the normal fluid above 1K and also allow vortex imaging in pure superfluid below 0.6 K [9]. Much progress has been made in developing high precision flow visualization techniques based on the use of He2* tracers. These techniques will foreseeably break new ground for various quantitative studies of high Re flows in cryogenic helium-4 as well as the quantum hydrodynamics in He II. A wide range of research and applications can benefit as illustrated in Fig. 2.
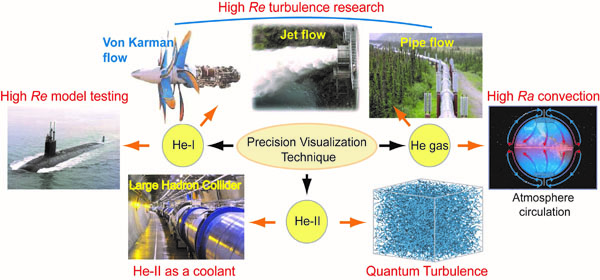
Figure 2: Examples of applications that can benefit from advanced flow visualization studies using cryogenic helium-4 as a test fluid.
Reference:
[1] R.J. Donnelly, "High Reynolds Number Flows Using Liquid and Gaseous Helium". (Springer-Verlag, New York, 1991).
[2] J.J. Niemela and K.R. Sreenivasan, "The use of cryogenic helium for classical turbulence: Promises and hurdles", J Low Temp Phys 143 (5-6), 163-212 (2006).
[3] L. Skrbek, J.J. Niemela, and R.J. Donnelly, "Turbulent flows at cryogenic temperatures: a new frontier", J Phys-Condens Mat 11 (40), 7761-7781 (1999).
[4] R.J. Donnelly, "Quantized vortices in helium II". (Cambridge University Press, Cambridge England; New York, 1991).
[5] W.F. Vinen and J.J. Niemela, "Quantum turbulence", J Low Temp Phys 129 (3-4), 213-213 (2002).
[6] W. Guo, M. La Mantia, D.P. Lathrop, and S.W. Van Sciver, "Visualization of two-fluid flows of superfluid helium-4 at finite temperatures", Proc Natl Acad Sci USA 111, 4653 (2014).
[7] G.P. Bewley, D.P. Lathrop, and K.R. Sreenivasan, "Superfluid helium - Visualization of quantized vortices", Nature 441 (7093), 588-588 (2006).
[8] W. Guo, S.B. Cahn, J.A. Nikkel, W.F. Vinen, and D.N. McKinsey, "Visualization study of counterflow in superfluid 4He using metastable helium molecules", Phys Rev Lett 105 (4), 045301 (2010).
[9] D.E. Zmeev, F. Pakpour, P.M. Walmsley, A.I. Golov, W. Guo, D.N. McKinsey, G.G. Ihas, P.V. McClintock, S.N. Fisher, and W.F. Vinen, "Excimers He2* as tracers of quantum turbulence in 4He in the t = 0 limit", Phys Rev Lett 110 (17), 175303 (2013).