Grid Turbulence Experiment

Despite being a two-fluid system, He II was observed to behave very similarly to classical fluids when a flow is produced by mechanical means that are conventionally used in classical fluid research, such as by a towed grid or a rotating propeller [1-2]. This similarity has brought up the feasibility of using He II for fundamental classical turbulence research and for model testing [3-4]. Many turbulent flows occurring in nature have extremely high Reynolds numbers, i.e. Re ~ 10⁸-10⁹, such as those generated by flying aircraft, rocket engine, and atmospheric convection. A grand challenge in classical fluid research is to produce these high Re flows for laboratory investigation. The Re number is proportional to the characteristic scale of the flow and inversely proportional to the fluid kinematic viscosity [5]. Therefore, a traditional route to increase Re is to construct expensive large-scale flow facilities and wind tunnels. But even with these facilities, it is still challenging to achieve Re over a few million using conventional fluid materials such as water and air. He II offers a new route to conduct high Re flow research in compact equipment due to its extremely small kinematic viscosity [3]. In our cryogenics lab, turbulent pipe flow in He II with Re ~ 2×10⁷ has been achieved [6].

The quasiclassical behaviour of He II in mechanically driven flows is believed to be the result of a strong coupling of the two fluids at large scales by mutual friction [7]. The turbulent eddies in the normal fluid are matched by eddies in the superfluid induced by local polarization of the vortex tangle [8]. At scales below the mean inter-vortex distance, this coupling breaks down because the superfluid flow is then dominated by the discrete vortex-line structure and cannot match any ordinary classical flow. Mutual friction dissipation sets in at these small scales. However, this important physical picture was indicated only indirectly from experimental measurement of the vortex-line density in the superfluid [1] or the pressure fluctuations resulted from mixed effects of both fluids [2]. Yet, recent numerical simulation raised the concern that strong correlations between the vorticity patterns in the two fluids may not exist [9]. Furthermore, it was suggested that the non-classical dissipation physics at small scales could alter many emergent flow properties such as effective viscosity and turbulent intermittency [10]. In order to lay a solid foundation for various exciting He II based turbulence applications, there is an imperative need to test the two-fluid coupling concept and to characterize the flow properties.

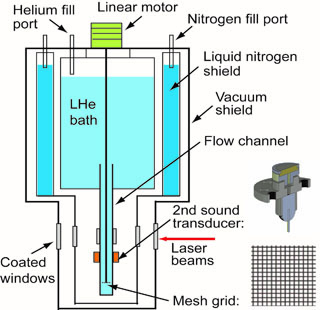
Figure 1: Schematic of the experimental setup for the towed-grid turbulence experiment.

We have launched a project to produce grid turbulence in He II and to study the emergent properties of this representative form of quasiclassical turbulence using tools that can independently probe the normal-fluid flow and the density of the vortices in the superfluid. Towed-grid generated turbulence is regarded as nearly homogeneous and isotropic, the study of which has played an important role in testing various theory models in classical turbulence research. To produce grid turbulence in helium, we will use a linear motor to pull a mesh grid through a He II filled channel in an optical cryostat, as shown in Fig. 1. This channel has a square cross section (side width 1.5 cm) and a length of 40 cm. The linear motor can pull the attached rod through the channel at a tunable speed U (1 mm/s to 1.2 m/s). We will probe the normal-fluid motion via flow visualization and will measure the vortex-line density L using a widely adopted method, called 2nd sound attenuation [11]. We aim to study the coupling of the two fluids by comparing the decay of turbulence energy in both fluids. We also plan to measure the structure functions and examine the intermittency in grid turbulence to see how the quantum nature of He-II could affect the properties of the quasiclassical flows in it. These pioneering studies will promote better applications of He-II in classical turbulence research and modelling applications.

Reference:

[1]   S.R. Stalp, L. Skrbek, and R.J. Donnelly, "Decay of grid turbulence in a finite channel", Phys Rev Lett 82 (24), 4831-4834 (1999).
[2]   J. Maurer and P. Tabeling, "Local investigation of superfluid turbulence", Europhys Lett 43 (1), 29-34 (1998).
[3]   L. Skrbek, J.J. Niemela, and R.J. Donnelly, "Turbulent flows at cryogenic temperatures: a new frontier", J Phys-Condens Mat 11 (40), 7761-7781 (1999).
[4]  K.R. Sreenivasan and R.J. Donnelly, "Role of cryogenic helium in classical fluid dynamics: Basic research and model testing", Adv Appl Mech 37, 239-276 (2001).
[5]   D.J. Tritton, Physical fluid dynamics. (Clarendon, Oxford, 1988), 2nd ed. [6]   S. Fuzier, B. Baudouy, and S.W. Van Sciver, "Steady-state pressure drop and heat transfer in HeII forced flow at high Reynolds number", Cryogenics 41 (5-6), 453-458 (2001).
[7]   W.F. Vinen, "Classical character of turbulence in a quantum liquid", Phys Rev B 61 (2), 1410-1420 (2000).
[8]   A.W. Baggaley, C.F. Barenghi, A. Shukurov, and Y.A. Sergeev, "Coherent vortex structures in quantum turbulence", Europhys Lett 98 (2), 26002 (2012).
[9]   D. Kivotides, "Interactions between normal-fluid and superfluid vortex rings in helium-4", Epl-Europhys Lett 112 (3) (2015).
[10]   L. Boue, V. L'vov, A. Pomyalov, and I. Procaccia, "Enhancement of Intermittency in Superfluid Turbulence", Phys Rev Lett 110 (1) (2013).
[11]   W. Zimmermann, "Porous-Membrane Second-Sound Transducers for Superfluid He-4", Phys Rev B 33 (1), 139-149 (1986).