(Panton3 23.5, 6, Tennekes & Lumley 4)
Maybe someday an Oracle of Delphi will arise that can actually model
turbulence accurately. Do not hold your breath.
Until then, the most solid way to learn some real stuff about
turbulence is without doubt dimensional analysis. Dimensional
analysis does not require you to actually solve the equations. It
just requires you to figure out what is important and what is not.
Some important examples follow.
Mixing layers:
Consider a turbulent mixing layer (AKA shear or vortex layer) as shown
above. Going downstream, you would expect the velocity profile to be
of the generic functional form
where  is the horizontal distance from the starting point of the
layer,
is the horizontal distance from the starting point of the
layer, 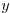 the vertical coordinate through the layer,
the vertical coordinate through the layer, 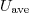 the average of the velocities above and below the layer, and
the average of the velocities above and below the layer, and 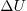 the velocity change over the layer.
the velocity change over the layer.
However, in a free turbulent flow, away from walls, the laminar shear
stress is small compared to the turbulent one. This suggests that the
laminar viscosity 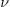 can be ignored in the relation above.
(Remember from the energy cascade discussion that the laminar stress
is important in the dissipation of turbulent kinetic energy. But it
should not be involved in the large-scale mechanics of the turbulent
mixing layer, like the instability mechanisms that sustain the big
eddies. If
can be ignored in the relation above.
(Remember from the energy cascade discussion that the laminar stress
is important in the dissipation of turbulent kinetic energy. But it
should not be involved in the large-scale mechanics of the turbulent
mixing layer, like the instability mechanisms that sustain the big
eddies. If 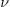 changes, it should only affect at which scales the
energy cascade gets rid of its energy.)
changes, it should only affect at which scales the
energy cascade gets rid of its energy.)
Also, if you look sufficiently far downstream, the details of the
initial conditions should no longer be visible.
Dimensional analysis can now be done for the mean velocity profile
 above. Selecting
above. Selecting  and
and 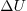 to
nondimensionalize the remaining variables gives
to
nondimensionalize the remaining variables gives
But the second argument of 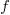 above is a constant, independent of
above is a constant, independent of  for a given mixing layer. So it follows that the mixing layer
velocity profile is similar, and that the layer has a typical
thickness
for a given mixing layer. So it follows that the mixing layer
velocity profile is similar, and that the layer has a typical
thickness 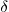 proportional to
proportional to  .
.
Exercise:
Discuss how well that seems to agree with flow visualizations.

Note that while in a boundary layer approximation the mixing layer can
reasonably be approximated as relatively thin compared to its
streamwise extend, this approximation does not improve with streamwise
distance.
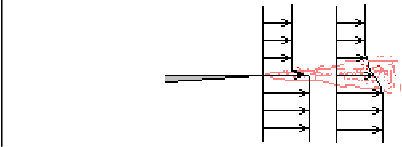
Jets:
Now consider a turbulent jet, as shown above. Here you would expect
that downstream
Again, 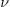 can be ignored, assuming that the turbulent Reynolds
number is large enough.
can be ignored, assuming that the turbulent Reynolds
number is large enough.
Also, it can again be assumed that the details of the initial
conditions become invisible sufficiently far downstream, with one
exception. Integral momentum conservation between any two downstream
positions of constant  implies that the
implies that the  -momentum flow integral
-momentum flow integral
must be the same at the two stations. (Pressure differences between
stations sufficiently far downstream can be ignored). So the momentum
flow integral above is a constant. It is determined by the strength
of the jet that the initial conditions generated. Since it is
constant, it cannot become invisible. Note that in the
incompressible case, you can more simply assume that
is constant. Also note that 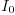 has units
has units 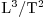 for a two
dimensional jet, where
for a two
dimensional jet, where 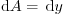 , but units
, but units 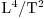 for a
three-dimensional jet, for which
for a
three-dimensional jet, for which 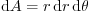 .
.
So the functional dependence can be simplified to
and dimensional analysis then produces
Cleaning this up gives:
Like for the mixing layer, the mean velocity profiles are similar, and
the jet thickness is proportional to  . But in the two-dimensional
case, the maximum jet velocity decays proportional to
. But in the two-dimensional
case, the maximum jet velocity decays proportional to  ,
slower than the
,
slower than the  of the three-dimensional case.
of the three-dimensional case.
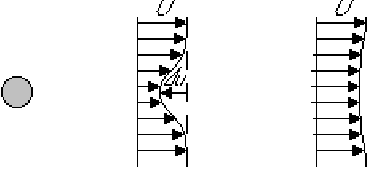
Wakes:
Finally consider the wake of some body, as shown above. Here you
would expect that downstream
Again, 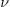 can be ignored. Also, it can again be assumed that the
details of the initial conditions become invisible sufficiently far
downstream, with the exception that
can be ignored. Also, it can again be assumed that the
details of the initial conditions become invisible sufficiently far
downstream, with the exception that
cannot become invisible because it is constant. If  has
become small enough, we can expand the square and ignore the
has
become small enough, we can expand the square and ignore the 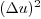 term to give that
term to give that
is constant. Since the first term is just a constant too, more simply
must be constant. In two dimensions this has units  and in three
and in three 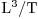 .
.
The functional relationship simplifies to
and dimensional analysis then gives
Because of the second argument of function 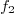 , there is little
useful knowledge that we can get from this.
, there is little
useful knowledge that we can get from this.
However, we can supplement the dimensional analysis with what we
believe to be true about the turbulence. Consider the two-dimensional
Reynolds-averaged  -momentum equation in boundary layer
approximation:
-momentum equation in boundary layer
approximation:
The average laminar stress should be negligible and when  has become small enough compared to
has become small enough compared to 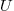 , we can also approximate the
left hand side to give
, we can also approximate the
left hand side to give
(Note that 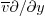 should, based on continuity,
be of the same order as
should, based on continuity,
be of the same order as 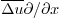 ,
hence negligible compared to the retained term.)
,
hence negligible compared to the retained term.)
If we ballpark the two sides in the simplified momentum equation
above,
or rearranged
In particular, there would be a problem in reasonably balancing the
momentum equation if 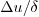 would be proportional to a
different power of
would be proportional to a
different power of  for large
for large  than
than 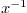 . In addition,
since
. In addition,
since 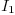 is constant, in two dimensions we also have the constraint
that
is constant, in two dimensions we also have the constraint
that 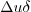 must stay of order
must stay of order 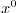 . Combining the two,
in two dimensions, as far as powers of
. Combining the two,
in two dimensions, as far as powers of  are concerned, we must have
that
are concerned, we must have
that
Now we trivially rewrite the previous relationship for  as
as
Here we have cleverly formed nondimensional combinations for  and
and 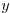 that should be independent of
that should be independent of  for large
for large  . But the
right hand side can be written as just a different function
. But the
right hand side can be written as just a different function 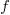 :
:
Function 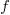 is not a new function, it is fully determined by
is not a new function, it is fully determined by 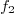 :
if you know the arguments of
:
if you know the arguments of 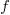 , you can compute those of
, you can compute those of 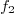 and
then
and
then 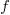 . But function
. But function 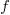 cannot depend on its second argument, or
else the shown nondimensional combinations for
cannot depend on its second argument, or
else the shown nondimensional combinations for  and
and 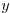 would not be independent of
would not be independent of  as they should.
as they should.
Cleaning up then gives
It may be noted that if substitute the above similar profile into the
simplified momentum equation above, assuming some suitable constant
eddy viscosity 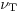 for the viscous term, you can find the
profile. You will get a reasonable approximation to the actual
measured wake velocity profile except near the outer edges. Note that
near the outer edges the flow is only part of the time truly
turbulent, as near the outer edges turbulent eddies engulf regions of
potential flow fluid. The
for the viscous term, you can find the
profile. You will get a reasonable approximation to the actual
measured wake velocity profile except near the outer edges. Note that
near the outer edges the flow is only part of the time truly
turbulent, as near the outer edges turbulent eddies engulf regions of
potential flow fluid. The intermittency
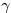 is
defined as the fraction of time that the flow is turbulent. It turns
out that if you assume that
is
defined as the fraction of time that the flow is turbulent. It turns
out that if you assume that 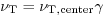 ,
with
,
with 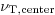 a suitable constant, you can get very good
agreement with the experimental profile.
a suitable constant, you can get very good
agreement with the experimental profile.
In three dimensions, accounting for the different 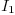 ,
,
Note that in this case, the Reynolds number based on  and
and
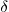 is proportional to
is proportional to  , so it decreases with
, so it decreases with  .
Therefore the made assumption of high Reynolds number will eventually
break down, and the wake will even become laminar. The result above
assumes that you do not look that far downstream. (The
two-dimensional wake above and the three-dimensional jet have Reynolds
numbers that stay constant with
.
Therefore the made assumption of high Reynolds number will eventually
break down, and the wake will even become laminar. The result above
assumes that you do not look that far downstream. (The
two-dimensional wake above and the three-dimensional jet have Reynolds
numbers that stay constant with  , so the approximation of high
Reynolds number, while qualitatively quite reasonable, does not
improve with
, so the approximation of high
Reynolds number, while qualitatively quite reasonable, does not
improve with  in those cases.)
in those cases.)
![]() can be ignored in the relation above.
(Remember from the energy cascade discussion that the laminar stress
is important in the dissipation of turbulent kinetic energy. But it
should not be involved in the large-scale mechanics of the turbulent
mixing layer, like the instability mechanisms that sustain the big
eddies. If
can be ignored in the relation above.
(Remember from the energy cascade discussion that the laminar stress
is important in the dissipation of turbulent kinetic energy. But it
should not be involved in the large-scale mechanics of the turbulent
mixing layer, like the instability mechanisms that sustain the big
eddies. If ![]() changes, it should only affect at which scales the
energy cascade gets rid of its energy.)
changes, it should only affect at which scales the
energy cascade gets rid of its energy.)
![]() above. Selecting
above. Selecting ![]() and
and ![]() to
nondimensionalize the remaining variables gives
to
nondimensionalize the remaining variables gives
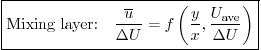

![]() implies that the
implies that the ![]() -momentum flow integral
-momentum flow integral
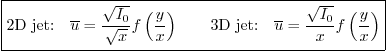
![]() can be ignored. Also, it can again be assumed that the
details of the initial conditions become invisible sufficiently far
downstream, with the exception that
can be ignored. Also, it can again be assumed that the
details of the initial conditions become invisible sufficiently far
downstream, with the exception that
![]() -momentum equation in boundary layer
approximation:
-momentum equation in boundary layer
approximation:
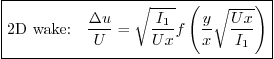
![]() for the viscous term, you can find the
profile. You will get a reasonable approximation to the actual
measured wake velocity profile except near the outer edges. Note that
near the outer edges the flow is only part of the time truly
turbulent, as near the outer edges turbulent eddies engulf regions of
potential flow fluid. The
for the viscous term, you can find the
profile. You will get a reasonable approximation to the actual
measured wake velocity profile except near the outer edges. Note that
near the outer edges the flow is only part of the time truly
turbulent, as near the outer edges turbulent eddies engulf regions of
potential flow fluid. The ![]() is
defined as the fraction of time that the flow is turbulent. It turns
out that if you assume that
is
defined as the fraction of time that the flow is turbulent. It turns
out that if you assume that ![]() ,
with
,
with ![]() a suitable constant, you can get very good
agreement with the experimental profile.
a suitable constant, you can get very good
agreement with the experimental profile.
![]() ,
,
![\begin{displaymath}
\fbox{$\displaystyle
\mbox{3D wake:}\quad
\frac{\Delta u}...
...x^2}}
f\left(\frac{y}{x}\sqrt[3]{\frac{Ux^2}{I_1}}\right)
$}
\end{displaymath}](img127.gif)