6 10/09 W

1. 5.12. This question explains why the water stream coming out of a faucet contracts in area immediately below the faucet exit. As always, both mass and momentum conservation are needed.

   The faucet exit velocity may be assumed to be of the form of Poisseuille flow:
   

   $$v_z = v_{\rm max} \left(1 - \frac{r^2}{R^2}\right)$$
   
   
   You can assume that the stress tensor at the faucet exit is of the form (in cylindrical coordinates)
   
   $$\bar{\bar\tau} = \left( \begin{array}{ccc} 0 & 0 & \tau_0 r/R 0 & 0 & 0 \tau_0 r/R & 0 & 0 \end{array} \right)$$
   
   
   in other words, much like the strain rate tensor that you derived earlier for Poisseuille flow.

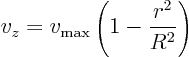
   Take the faucet exit as the entrance of your control volume. Take as exit to your control volume a slighly lower plane at which the radius of the jet has stabilized to $R_2$ and the flow velocity has become uniform (independent of r). For a uniform flow velocity there are no viscous stresses. Gravity can be ignored compared to the high viscous forces in this very viscous fluid. (However, over a longer distance gravity will lead to a further thinning of the jet.) And you can assume that the pressure at the exit is already atmospheric, as it definitely is in the lower plane below.

2. Write a finite volume discretization for the $x$-momentum equation for the little finite volume in polar coordinates. Just like the continuity equation done in class, your final equation should only involve pressures, densities, and velocities at the center points of the finite volumes. Ignore the viscous stresses for now.

   The unknown velocities used in the computation should be taken to be the polar components $v_r$ and $v_\theta$. But momentum conservation for $x$-momentum is asked. (Conservation of $r$-momentum or $\theta$-momentum would be complete nonsense.) So you will need to write the $x$-component of velocity in terms of the polar unknowns. Note that in Cartesian coordinates, the polar unit vectors are given by
   

   $${\widehat \imath}_r=\cos(\theta){\widehat \imath}+ \sin(\th... ... \sin(\theta){\widehat \imath}+ \cos(\theta){\widehat \jmath}$$
   
   
   These should be able to allow you to evaluate the $x$-components of velocity and pressure forces that you need.

3. Assuming that there are known viscous stress tensors at the centers of the sides of the finite element, what additional terms do you get in the obtained equation due to viscous forces? Assume the stress tensor is given in polar form. (So $\tau_{rr}$, $\tau_{r\theta}$, etcetera.) Once again you will need the polar unit vectors to get the $x$-components of the forces.