5.6 Iden­ti­cal Par­ti­cles

A num­ber of the counter-in­tu­itive fea­tures of quan­tum me­chan­ics have al­ready been dis­cussed: Elec­trons be­ing nei­ther on Mars or on Venus un­til they pop up at ei­ther place. Su­per­lu­mi­nal in­ter­ac­tions. The fun­da­men­tal im­pos­si­bil­ity of im­prov­ing the ac­cu­racy of both po­si­tion and mo­men­tum be­yond a given limit. Col­lapse of the wave func­tion. A hid­den ran­dom num­ber gen­er­a­tor. Quan­tized en­er­gies and an­gu­lar mo­menta. Nonex­ist­ing an­gu­lar mo­men­tum vec­tors. In­trin­sic an­gu­lar mo­men­tum. But na­ture has one more trick on its sleeve, and it is a big one.

Na­ture en­tan­gles all iden­ti­cal par­ti­cles with each other. Specif­i­cally, it re­quires that the wave func­tion re­mains un­changed if any two iden­ti­cal bosons are ex­changed. If par­ti­cles $i$ and $j$ are iden­ti­cal bosons, then:

$$\Psi\left({\skew0\vec r}_1, S_{z1}, \ldots, {\skew0\vec r}... ... r}_j, S_{zj}, \ldots, {\skew0\vec r}_i, S_{zi}, \ldots\right)$$ (5.27)

On the other hand, na­ture re­quires that the wave func­tion changes sign if any two iden­ti­cal fermi­ons are ex­changed. If par­ti­cles $i$ and $j$ are iden­ti­cal fermi­ons, (say, both elec­trons), then:

$$\strut\Psi\left({\skew0\vec r}_1, S_{z1}, \ldots, {\skew0\v... ... r}_j, S_{zj}, \ldots, {\skew0\vec r}_i, S_{zi}, \ldots\right)$$ (5.28)

In other words, the wave func­tion must be sym­met­ric with re­spect to ex­change of iden­ti­cal bosons, and an­ti­sym­met­ric with re­spect to ex­change of iden­ti­cal fermi­ons. This greatly re­stricts what wave func­tions can be.

For ex­am­ple, con­sider what this means for the elec­tron struc­ture of the hy­dro­gen mol­e­cule. The ap­prox­i­mate ground state of low­est en­ergy was in the pre­vi­ous sec­tion found to be

$$\psi_{\rm {gs}} = a \left[ \psi_{\rm {l}}({\skew0\vec r}_... ...ownarrow}{\uparrow}+ a_{-}{\downarrow}{\downarrow} \right] %$$ (5.29)

were $\psi_{\rm {l}}$ was the ground state of the left hy­dro­gen atom, $\psi_{\rm {r}}$ the one of the right one, first ar­rows in­di­cate the spin of elec­tron 1 and sec­ond ar­rows the one of elec­tron 2, and $a$ and the $a_{\pm\pm}$ are con­stants.

But since the two elec­trons are iden­ti­cal fermi­ons, this wave func­tion must turn into its neg­a­tive un­der ex­change of the two elec­trons. Ex­chang­ing the two elec­trons pro­duces

$$- \psi_{\rm {gs}} = a \left[ \psi_{\rm {l}}({\skew0\vec r... ...{\uparrow}{\downarrow}+ a_{-}{\downarrow}{\downarrow}\right];$$

note in par­tic­u­lar that since the first ar­row of each pair is taken to re­fer to elec­tron 1, ex­chang­ing the elec­trons means that the or­der of each pair of ar­rows must be in­verted. To com­pare the above wave func­tion with the nonex­changed ver­sion (5.29), re­order the terms back to the same or­der:

$$- \psi_{\rm {gs}} = a \left[ \psi_{\rm {l}}({\skew0\vec r... ...}{\downarrow}{\uparrow}+ a_{-}{\downarrow}{\downarrow}\right]$$

The spa­tial fac­tor is seen to be the same as the nonex­changed ver­sion in (5.29); the spa­tial part is sym­met­ric un­der par­ti­cle ex­change. The sign change will have to come from the spin part.

Since each of the four spin states is in­de­pen­dent from the oth­ers, the co­ef­fi­cient of each of these states will have to be the neg­a­tive of the one of the nonex­changed ver­sion. For ex­am­ple, the co­ef­fi­cient $a_{++}$ of ${\uparrow}{\uparrow}$ must be the neg­a­tive of the co­ef­fi­cient $a_{++}$ of ${\uparrow}{\uparrow}$ in the nonex­changed ver­sion, oth­er­wise there is a con­flict at $S_{z1}$ $\vphantom0\raisebox{1.5pt}{$=$}$ $\frac12\hbar$ and $S_{z2}$ $\vphantom0\raisebox{1.5pt}{$=$}$ $\frac12\hbar$, where only the spin state ${\uparrow}{\uparrow}$ is nonzero. Some­thing can only be the neg­a­tive of it­self if it is zero, so $a_{++}$ must be zero to sat­isfy the an­ti­sym­me­try re­quire­ment. The same way, $a_{-}$ $\vphantom0\raisebox{1.5pt}{$=$}$ $-a_{-}$, re­quir­ing $a_{-}$ to be zero too. The re­main­ing two spin states both re­quire that $a_{+-}$ $\vphantom0\raisebox{1.5pt}{$=$}$ $-a_{-+}$, but this can be nonzero.

So, due to the an­ti­sym­metriza­tion re­quire­ment, the full wave func­tion of the ground state must be,

$$\strut\strut\psi_{\rm {gs}} = a \left[ \psi_{\rm {l}}({\s... ...} \left[ {\uparrow}{\downarrow}- {\downarrow}{\uparrow}\right]$$

or af­ter nor­mal­iza­tion, not­ing that a fac­tor of mag­ni­tude one is al­ways ar­bi­trary,

$$\psi_{\rm {gs}} = a \left[ \psi_{\rm {l}}({\skew0\vec r}_... ... \frac{{\uparrow}{\downarrow}- {\downarrow}{\uparrow}}{\sqrt2}$$

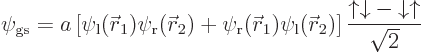
It is seen that the an­ti­sym­metriza­tion re­quire­ment re­stricts the spin state to be the sin­glet one, as de­fined in the pre­vi­ous sec­tion. It is the sin­glet spin state that achieves the sign change when the two elec­trons are ex­changed; the spa­tial part re­mains the same.

If the elec­trons would have been bosons, the spin state could have been any com­bi­na­tion of the three triplet states. The sym­metriza­tion re­quire­ment for fermi­ons is much more re­stric­tive than the one for bosons.

Since there are a lot more elec­trons in the uni­verse than just these two, you might rightly ask where an­ti­sym­metriza­tion stops. The an­swer given in chap­ter 8.3 is: nowhere. But don’t worry about it. The ex­is­tence of elec­trons that are too far away to af­fect the sys­tem be­ing stud­ied can be ig­nored.

Key Points

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The wave func­tion must be sym­met­ric (must stay the same) un­der ex­change of iden­ti­cal bosons.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The wave func­tion must be an­ti­sym­met­ric (must turn into its neg­a­tive) un­der ex­change of iden­ti­cal fermi­ons (e.g., elec­trons.)

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

Es­pe­cially the an­ti­sym­metriza­tion re­quire­ment greatly re­stricts what wave func­tions can be.

$\begin{picture}(15,5.5)(0,-3) \put(2,0){\makebox(0,0){\scriptsize\bf0}} \put(12... ...\thicklines \put(3,0){\line(1,0){12}}\put(11.5,-2){\line(1,0){3}} \end{picture}$

The an­ti­sym­metriza­tion re­quire­ment forces the elec­trons in the hy­dro­gen mol­e­cule ground state to as­sume the sin­glet spin state.

5.6 Re­view Ques­tions

1.

Check that in­deed any lin­ear com­bi­na­tion of the triplet states is un­changed un­der par­ti­cle ex­change.

So­lu­tion ident-a

2.

Sup­pose the elec­trons of the hy­dro­gen mol­e­cule are in the ex­cited an­ti­sym­met­ric spa­tial state

$$a \left[ \psi_{\rm {l}}({\skew0\vec r}_1)\psi_{\rm {r}}({\sk... ...r}}({\skew0\vec r}_1)\psi_{\rm {l}}({\skew0\vec r}_2) \right].$$

In that case what can you say about the spin state?

Yes, in this case the spin would be less re­stricted if the elec­trons were bosons. But an­ti­sym­met­ric spa­tial states them­selves are pretty re­stric­tive in gen­eral. The pre­cise sense in which the an­ti­sym­metriza­tion re­quire­ment is more re­stric­tive than the sym­metriza­tion re­quire­ment will be ex­plored in the next sec­tion.

So­lu­tion ident-b