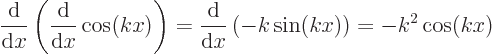2.5.2 So­lu­tion eigvals-b

Ques­tion:

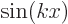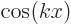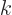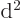
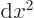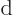
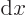Show that any func­tion of the form $\sin(kx)$ and any func­tion of the form $\cos(kx)$, where $k$ is a con­stant called the wave num­ber, is an eigen­func­tion of the op­er­a­tor ${\rm d}^2$$\raisebox{.5pt}{$/$}$​${\rm d}{x}^2$, though they are not eigen­func­tions of ${\rm d}$$\raisebox{.5pt}{$/$}$​${\rm d}{x}$.

An­swer:

The first de­riv­a­tives are, [1, p. 60]:

$$\frac{{\rm d}}{{\rm d}x}\sin(kx) = k \cos(kx) \qquad\frac{{\rm d}}{{\rm d}x}\cos(kx) = - k \sin(kx)$$

so they are not eigen­func­tions of 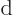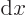${\rm d}$$\raisebox{.5pt}{$/$}$​${\rm d}{x}$. But a sec­ond dif­fer­en­ti­a­tion gives:

$$\frac{{\rm d}}{{\rm d}x}\left(\frac{{\rm d}}{{\rm d}x}\sin(k... ...rac{{\rm d}}{{\rm d}x}\left(k \cos(kx)\right) = - k^2 \sin(kx)$$

$$\frac{{\rm d}}{{\rm d}x}\left(\frac{{\rm d}}{{\rm d}x}\cos(k... ...ac{{\rm d}}{{\rm d}x}\left(-k \sin(kx)\right) = - k^2 \cos(kx)$$