| Analysis in Mechanical Engineering |
|
© Leon van Dommelen |
|
Subsections
1.2 Example
From [1, p. 128, 13a]
Asked: Draw the graph of
1.2.1 Using reasoning
Instead of starting to crunch numbers, look at the pieces first:
Factor 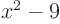

 is a parabola with zeros at
is a parabola with zeros at 

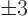 :
:
Squaring gives a quartic with double zeros at 

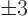 :
:
Dividing by  will produce a simple pole at
will produce a simple pole at 
 0 and also a sign
change at negative
0 and also a sign
change at negative  :
:
Function 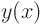 :
:
- has an
 -extent
-extent  and a
and a 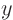 -extend
-extend 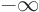
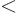
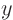
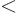
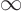 ;
;
- is odd (symmetric with respect to the origin);
- has a relative maximum at -3 of finite curvature:
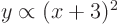 ;
;
- has a relative minimum at 3 of finite curvature:
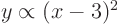 ;
;
- has a vertical asymptote at

 0 with asymptotic behavior:
0 with asymptotic behavior:
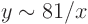 for
for 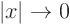 ;
;
- behaves asymptotically as
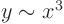 for
for 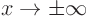 ;
;
- is concave up for

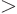 0, down for
0, down for 
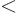 0. (Should really prove
this, I guess.)
0. (Should really prove
this, I guess.)
1.2.2 Using brute force
Hence
- intercepts with
 -axis are at
-axis are at 

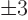 ;
;
- no intercepts with the
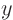 axis;
axis;
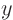 is an odd function of
is an odd function of  (symmetric about the origin);
(symmetric about the origin);
- for
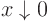 ,
, 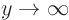 (vertical asymptote);
(vertical asymptote);
- for
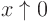 ,
,  (singularity is an odd, simple pole);
(singularity is an odd, simple pole);
- for
 ,
, 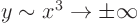 .
.
Hence,
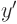
 0 for
0 for 
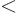

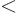
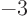 (
(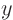 increases
from
increases
from 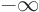 );
);
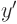
 0 for
0 for 

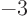 (local maximum,
(local maximum, 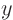
 0);
0);
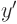
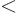 0 for
0 for 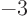
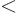

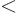 0 (
0 (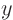 decreases towards
decreases towards 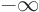 );
);
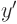

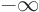 for
for 
 0 (singular point, vertical asymptote);
0 (singular point, vertical asymptote);
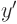
 0 for 0
0 for 0 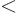

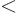
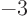 (decreases from
(decreases from 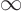 );
);
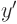
 0 for
0 for 
 3 (local minimum,
3 (local minimum, 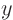
 0);
0);
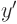
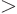 0 for 3
0 for 3 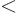

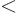
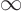 (increases to
(increases to 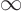 ).
).
Also,
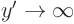 when
when  (no horizontal or oblique
asymptotes);
(no horizontal or oblique
asymptotes);
- all derivatives exist, except at

 0, which has no point
on the curve (no corners, cusps, infinite curvature, or other
singular points);
0, which has no point
on the curve (no corners, cusps, infinite curvature, or other
singular points);
- probably no inflection points.
Hence
- really no inflection points (since there is no point at

 0);
0);
- cocave downward for

 0, upward for
0, upward for 
 0.
0.
Hence the  - and
- and 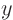 -extends are as before.
-extends are as before.
![]()
![]()
![]() is a parabola with zeros at
is a parabola with zeros at ![]()
![]()
![]() :
:
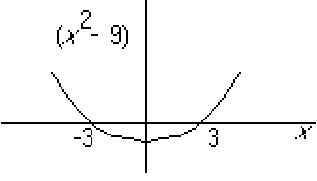
![]()
![]()
![]() :
:
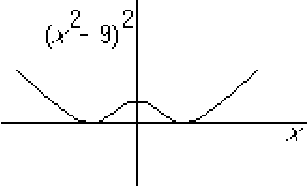
![]() will produce a simple pole at
will produce a simple pole at ![]()
![]() 0 and also a sign
change at negative
0 and also a sign
change at negative ![]() :
:
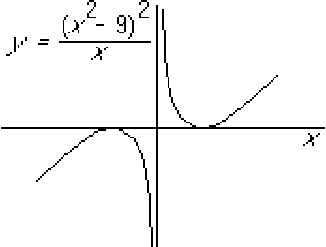
![]() :
:
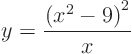
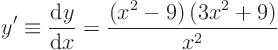
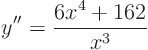
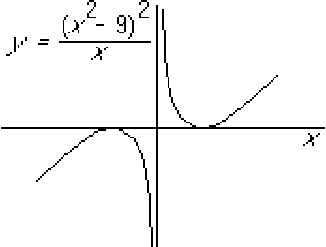
![]() - and
- and ![]() -extends are as before.
-extends are as before.