5.2 Example

From [1, p. 422, 27a]

Given:

$$\omega = \sqrt[3]{\frac gb}$$

The maximum error in $g$ is $1\%$, the maximum error in $b$ is $0.5\%$.

Asked: The maximum percentage error in $\omega$.

5.2.1 Identification

Given is that the maximum error in $g$ is $1\%$ and the maximum error in $b$ is $0.5\%$. That means that the relative errors are:

$$\frac{\delta g}g \le 0.01 \qquad \frac{\delta b}b \le 0.005$$

where $\delta g$ and $\delta b$ are the absolute errors. Errors are always positive.

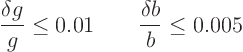
Error manipulation rules:

1.

During addition and substraction of variables, add their absolute errors;

2.

During multiplication or division, add their relative errors;

3.

During exponentiation, multiply the relative error by the absolute power.

5.2.2 Results

Consider first the relative change in $g/b$ due to changes ${\rm d}g$ in $g$ and ${\rm d}b$ in $b$. The rule for differentiating a ratio implies:

$$\frac{{\rm d}(g/b)}{(g/b)} = \frac{b}{g} \left( \frac{b ... ...\rm d}b}{b^2} \right) = \frac{{\rm d}g}g - \frac{{\rm d}b}b$$

Note that if you do not know the sign of the errors, you can only say that the final result is no bigger than

$$\left\vert\frac{{\rm d}g}g\right\vert + \left\vert\frac{{\rm d}b}b\right\vert$$

which is simply the rule for adding relative errors if you take a ratio or product of variables.

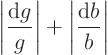
Hence the greatest possible relative error in $(g/b)$ is:

$$\frac{\delta (g/b)}{(g/b)} = 0.01 + 0.005 = 0.015$$

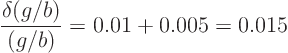
But we need the relative error in $\sqrt[3]{g/b}$ instead of in $g/b$. Denoting $g/b$ by $u$ for now, the rule for differentiating a power gives

$$\frac{{\rm d}u^{1/3}}{u^{1/3}} = \frac{\frac13 u^{-2/3} {\rm d}u}{u^{1/3}} = {\textstyle\frac{1}{3}} \frac{{\rm d}u}{u}$$

That is simply the rule of multiplying the relative error by the absolute power when exponentiating.

Hence

$$\frac{\delta\omega}\omega = {\textstyle\frac{1}{3}} \times 0.015 = 0.005 = 0.5\%$$